
Horizontal component of velocity × (Total flight time) which gives OA ux / t. The equation for projectile motion is y ax + bx2. The angle of the trajectory in a given point is the same as the angle that the velocity vector form with the horizontal at that point. The common formula for calculating a horizontal range is: Horizontal range. Once the strong> flight time is obtained, simply substitute in the equation of position of the horizontal component. It is the maximum horizontal distance, from the starting point of the motion to the point in which the body hits the ground. On the other hand, projectile motion is motion in. That is, the flight time is the time required for the height to become 0 (the projectile reaches the ground). When an object moves in one dimension, whether vertical or horizontal, we refer to it as a linear motion.

It is calculated for y = 0, the vertical component of the position. From that time, and from the equations of position, we can calculate the distance to the origin in the both axes, the x-axis and y-axis. Substitute t by 2 V0 sin () / g and simplify to obtain the range AC. range AC x V0 cos () t at t time of flight 2 V0 sin () / g. Starting from the equation of velocity in the y-axis, and making v y = 0, we get the time t that it takes the body in get to this height. Horizontal Range of a Projectile (distance AC in the figure above) Distance AC which is the horizontal range is equal to x when t is equal to the time of flight 2 V0 sin () / g obtained above. This value is reached when the velocity in the y-axis, v y , is 0. The projectile-motion equation is s(t) gx2 + v0x + h0, where g is the constant of gravity, v0 is the initial velocity (that is, the velocity at time t. The launch velocity of a projectile can be calculated from the range if the. On the other hand, frequently in exercises, you would be asked for some of the following values. The basic motion equations can be solved simultaneously to express y in. Examples of two-dimensional motion are as. This is the equation of projectile motion it is similar to the parabola( y ax + bx 2)so we can say that projectile motion is always parabolic in nature. The equation is: y x tan gx 2 /2u 2 cos 2. On the other hand, to know which trajectory the body follows, that is, its equation of trajectory, we can combine the above equations to eliminate t, getting:Īs expected, this is the equation of a parabola. Equation of the trajectory is a path followed by the particle during the projectile motion.

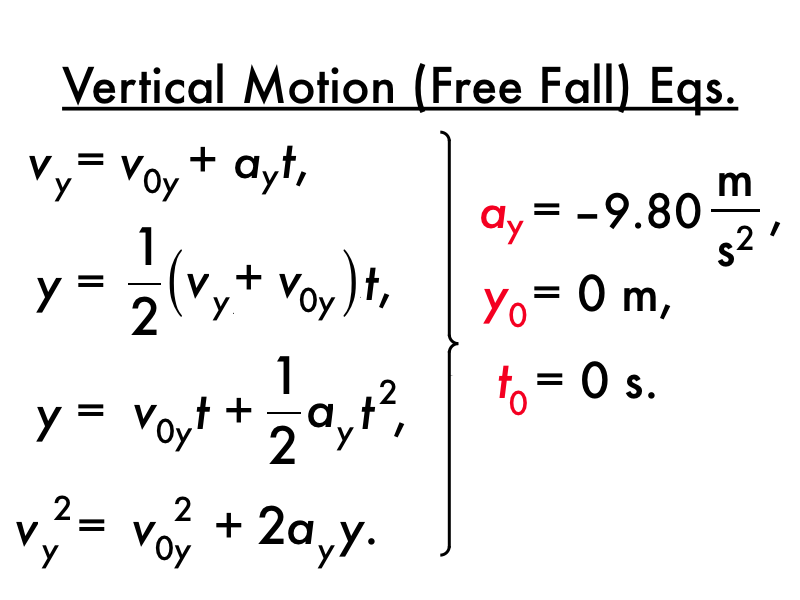
The equation of position of the projectile motion is given by:


 0 kommentar(er)
0 kommentar(er)
